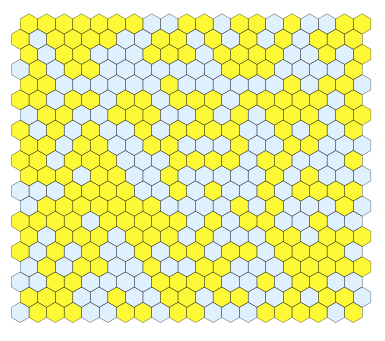
Imagine that you tossed an equal number of black and white balls into a rectangular box.  What would be the probability that a row of consecutive white balls would stretch from one end to the other? This question, posed in 1894 in the first issue of American Mathematical Monthly (along with an incorrect solution) is perhaps one of the earliest known examples of percolation theory, a rich and complicated mathematical field with applications to statistical and theoretical physics.

In 2001, Stanislav Smirnov caught the attention of the mathematics world when he solved one of the most elusive problems in percolation, validating assumptions physicists had long held but could not rigorously prove. In the process, he formed new theories that placed a firm foundation underneath the burgeoning world of mathematical physics. His discoveries earned him the Fields Medal, the highest honor in mathematics, in 2010.

Born in Russia, Smirnov came to Caltech in 1992, recruited by Nikolai Makarov, now the Richard Merkin Distinguished Professor of Mathematics. “Early on, he distinguished himself as a brilliant young mathematician,” Makarov said. “So I convinced him to come with me.”

“It was a complicated time for Russia,” Smirnov recalled. “The Soviet Union had just collapsed, and there was a great deal of uncertainty for everyone, especially for academic sciences—which suddenly felt cut off. Caltech felt like a world away, and an adventure.”

After earning his degree, Smirnov held research positions at a number of universities, eventually moving to the Royal Institute of Technology in Stockholm, where he dedicated himself to statistical physics, and percolation theory in particular. 

Education

• BSc, MSc, Mathematics, St. Petersburg State University, Russia, 1992
• MS, 1995, PhD, 1996, Mathematics, Caltech

Caltech Thesis
Spectral analysis of Julia sets, 1996

Current Position
Professor of Mathematics, University of Geneva; Director of SwissMAP, National Centre of Competence in Research

Selected Awards

• Salem Prize, 2001
• Clay Research Award, 2001
• European Mathematical Society Prize, 2004
• Fields Medal, 2010

Fields Medal Citation
"For the proof of conformal invariance of percolation and the planar Ising model in statistical physics"

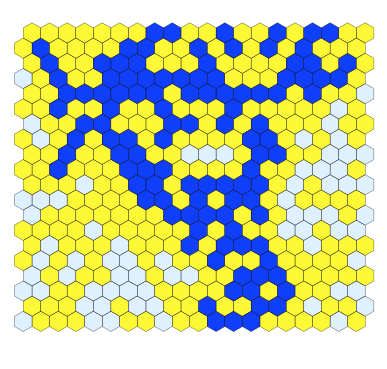
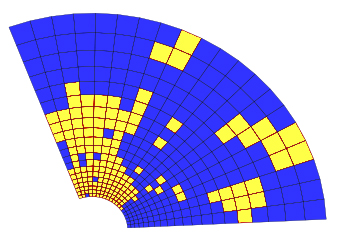
Though highly theoretical, percolation relates to a number of practical questions. Instead of the path through a box of balls, imagine liquid pouring through a porous material such as a sponge (mathematicians use the term “lattice”). What is the probability that the liquid will be able to wind its way down the system of holes through the lattice to finally reach the bottom? 

This is referred to as a crossing probability, and there is a particular critical point. Below it, the density of holes will not let liquid through, but above, the system suddenly flips behavior, allowing liquid to flow freely throughout. “We call such sudden flips phase transitions,” Smirnov said. “These simple models can be used to study ones we see in nature, such as when water boils to become steam, or the moment a piece of iron, when heated, stops being magnetic.”

There was, however, a looming mystery. Theoretical physicists were curious about what happened as you went down to the smallest possible scale, where the holes got smaller and smaller until their size reached zero, otherwise known as the scaling limit. Could you determine the crossing probability at such a scale? The answer had many implications, including for quantum field theory. One physicist, John Cardy, theorized a formula to calculate it in 1992. But no one could prove that such a value would even exist, much less if Cardy’s formula was accurate.

“Mathematics is the language of physics,” said Makarov. “Physicists observed these phenomena and thought they understood how these systems worked, but they lacked the mathematical evidence.”

Then, in 2001, Smirnov announced a breakthrough. Employing a series of novel and elegant techniques, he proved the crossing probability existed for the scaling limit for a two-dimensional triangular lattice—and that, indeed, it was equal to the value calculated by Cardy's formula. As a follow-up, Smirnov applied his techniques to other problems, including a famous one relating to ferromagnetism named after physicist Ernst Ising.

     “Increasingly we see mathematics playing a greater role within a number of fields…it truly is the universal language.”    

“You could go to the library and find shelves of books and papers working on these and similar problems over a hundred years,” said Makarov. “None of them had precise results until Smirnov. He nailed it. The significance of it cannot be overstated.”

Smirnov’s discovery opened the door for more interdisciplinary research across math and physics. Today, he directs a new center specializing in that intersection, the Swiss National Centre of Competence in Research (SwissMAP), and is a professor at the University of Geneva.

“Mathematics was for a time perhaps a very specialized field,” Smirnov said. “Increasingly we see it playing a greater role within a number of fields, including physics, biology, economics, and computer science…it truly is the universal language.”

by Ben Tomlin
Photo: Jon Rou

Slipping Through Even the Smallest Cracks

Smirnov's signature achievements relate to percolation theory, a rich mathematical field with applications to statistical and theoretical physics.